The property of a body by virtue of which, it opposes the torque tending to change its state of rest or of uniform rotation about an axis is called rotational inertia or moment of inertia.
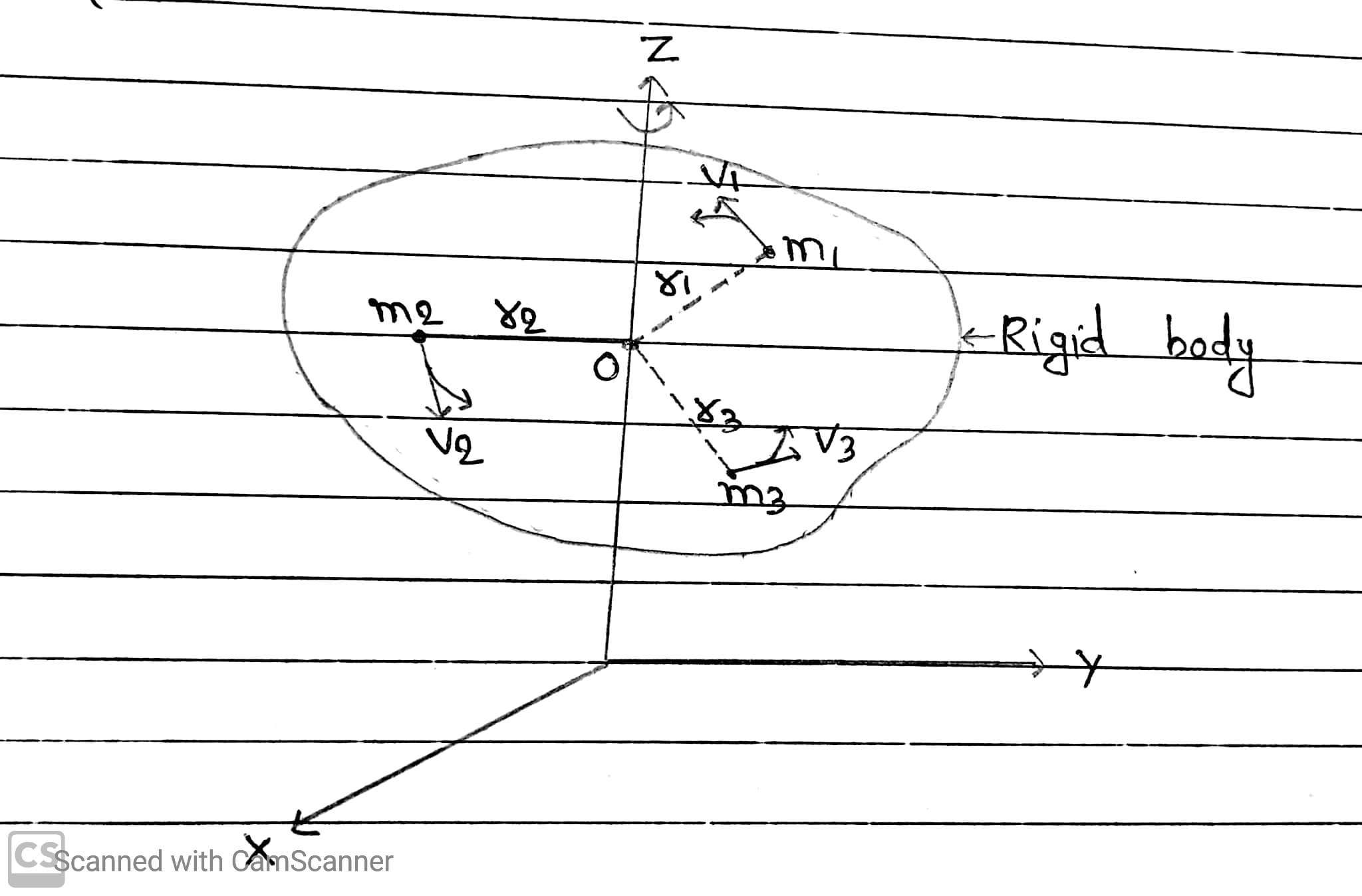
Let a rigid body rotating with uniform angular velocity ω about a vertical axis through O. Let the body consists of n particles of masses m₁, m₂, m₃,……mₙ situated at distances r₁, r₂, r₃, …….rₙ respectively from the axis of rotation.
The moment of inertia of the body about the axis OZ is given by

The SI unit of moment of inertia is kgm² and dimensional formula is [ML²T⁰].
Hence, moment of inertia of a rigid body about a fixed axis is defined as the sum of the products of the masses of the particle constituting the body and the squares of their respective distenses from the axis of rotation.
